Hotelling’s Model of Spatial Competition

Harold Hotelling in his research paper published in 1929 titled “Stability in Competition” proposed an economic model that helps in determining the equilibrium condition in duopolistic markets. The idea behind the model was to illustrate how consumers’ purchasing decisions depend not just on the characteristics of a product and its price but also the location of the sellers. A seller may, in effect, sell at a greater price than others, but still enjoy the same demand and the inflow of the same number of consumers, if she is located at a convenient position. A factor that will greatly determine the equilibrium in this model is the cost of travel, which is directly proportional to the distance between the consumer and the firm, and depends on no other factor (an assumption). Furthermore, a consumer might not be willing to travel a greater distance to acquire a product, even if the firm closest to the consumer sells at a price greater than the others. Other assumptions implicitly mentioned by Hotelling include - a) each consumer has unit demand, b) consumers are equitably distributed, c) cost of travelling will be determined by the same formula for each consumer, d) the firms incur zero or equal production costs and e) firms are perfectly mobile and cost of relocation is zero. It is to be noted that in Hotelling’s model, consumer demand corresponding to each firm will depend entirely upon the distance between the firm and the consumer, and no other factor, owing to the assumptions specified above. For this purpose, a scenario was created wherein a homogeneous product will exist, sold by two firms (with no alternative competition) at the same price. A horizontal line segment will indicate several location-setting sub-games, and at the end, we will be able to determine how the two firms will finally settle in a position of equilibrium.

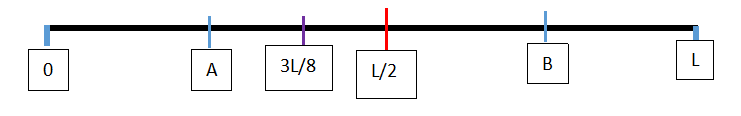
Let the two firms be A and B. The only available location for setting up their establishments is along a horizontal straight-lined street. Let the length of the street be represented by the variable L. Let the starting point be assumed as the left end of the street. The ideal position would be at a distance of L/4 from either end of the street for each firm, as illustrated by the diagram below.

The above diagram would ideally represent an equilibrium condition, wherein each firm equally shares the demand, since consumers to the left of the mid-point (L/2) will purchase from A and those on the right will purchase from B. Furthermore, consumers have to travel the least distance to purchase the product in this condition.

Hotelling notes that this sub-game cannot exist in the long-run, since in the absence of tacit collusion, one of the firms will definitely relocate to a different point to acquire the larger share of the market. In this case, let us assume that the owner of firm B decides to move the firm to the mid-point of the street. She realises that by employing this strategy, she can increase the effective demand of the product to include consumers from the right side of the mid-point (as was the case before) and those to the right side of the point located at a distance of 3L/8 from the mid-point. This effectively means that B will get all the consumers along a distance of 5L/8, and A along that of 3L/8. In other words, B’s demand will be greater than A’s. This is the second sub-game disequilibrium situation.

In order to acquire her demand back, the only strategy that can be adopted by A is to transfer her firm to the mid-point (L/2). This forms the third sub-game and in fact the equilibrium condition in Hotelling’s Model of Spatial Competition. Both firms will be located at the mid-point of the horizontal street. For convenience, we can assume that they are located right next to each other on either side of the mid-point, and both A and B will acquire an equal share of the market (same as the first sub-game). It is to be noted that neither firm can benefit from relocating and hence this will technically be the point of equilibrium. While this is the point of equilibrium, the consumer doesn’t benefit in this situation. The travel distance and hence the cost of the product will increase. This point is also the Nash Equilibrium[I].

When firms can alter prices

Let us explore an avenue which comes closer to reality than that proposed by Hotelling. Let us assume that prices are not constant. Let us analyse the possibilities in each of the sub-games mentioned above:

1) First sub-game: We know that for the consumer, the cost of the product is actually its price plus commute costs. If any firm - A or B changes her price, they will lose some of their demand but not all, as opposed to Bertrand’s Model[1]. The consumers located sufficiently close to a firm that has augmented its price will still buy the product from that firm, owing to the low transportation costs. Hence, a clever enterprise will regulate the price so as to reach a maximum profit situation by

analysing price-quantity relationship in the market.

2) Second sub-game: This will be very similar to the first sub-game, with the exception that B will have more freedom to adjust her price owing to her acquiring the majority demand.

3) Third sub-game: This being the Nash Equilibrium, the firms cannot move to any other location which will benefit them. The consumers at any point on the horizontal line-segment can purchase from either firm. Hence, any change in price by either firm will lead to the respective firm either rescinding or acquiring the entire demand. For example, if B decides to reduce her price, then the entire demand will be transferred to B and A’s demand will fall to 0, since the consumer is indifferent between A and B w.r.t. location. Hence, this price war will continue between A and B, each under-cutting the other’s price by some amount. The question is: When will it end? The answer lies in the Bertrand Paradox], which states that in the existence of high levels of competition and the absence of tacit collusion, firms charge a price equal to the marginal cost, which reduces their super-profits to zero. An identical situation will exist here and firms will continue to undercut each other until they reach a situation of zero super-normal profit. Any reduction of the price below this level by, say, firm B will lead to her incurring losses and A breaking even, which is absurd. However, firm B can continue incurring losses to a point where A exits the market for lack of demand and B finds herself to be the monopolist. But that’s a different discussion, and beyond the scope of this discourse. We have hence established that at the Nash Equilibrium point, Bertrand Paradox will operate.

Hence, we have now understood how price-determination works in Hotelling’s Model.

What if firms form cartels?

In a duopolistic condition, tacit collusion often happens and this results in the formation of cartels. Firms decide a price among themselves and sell their products at that specific price. The consumer is exploited in this situation and for a firm, there is a risk of the other breaching the agreement.

Hotelling, hence explained in 1929 the reason why shopping malls exist, with ten food-joints located within a radius of one hundred meters and still manage to exploit the consumer in an increasingly capitalistic world.

Definitions:

I. Nash Equilibrium: A stable state of a system involving the interaction of different participants, in which no participant can gain by a unilateral change of strategy if the strategies of the others remain unchanged.

II. Bertrand’s Paradox: A situation in which two players (firms) reach a state of Nash equilibrium where both firms charge a price equal to marginal cost ("MC").

References:

1. https://www.economicsdiscussion.net/oligopoly/bertrands-duopoly-model-with-diagram/5396

Further Readings:

1. Two-Stage (Perfect) Equilibrium in Hotelling's Model: Damien Neven (1985)

2. On Hotelling's "Stability in Competition": C. d’Aspremont et al (1979)

3. Equilibrium in Hotelling's Model of Spatial Competition: Martin J. Osborne and Carolyn Pitchik (1987)

4. Stability in Competition: Harold Hotelling (1929)

- Soumil Agarwal

soumilagarwal@gmail.com